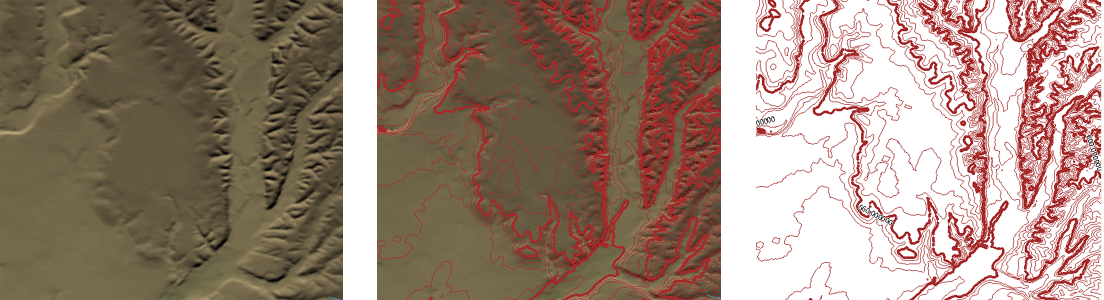
Contours are imaginary lines on the map which join points on the earth's surface at the same elevation. Contour lines are measured from a base datum, which is usually sea level, You can also have lines measured down from the datum to show the contours of the ocean floor or other bodies of water. Contour lines have become a familiar convention for representing elevations on large-scale maps, such as topographic maps, where accuracy and readability are more important than visual effect.
In AutoCAD Map 3D, you can create contours from surfaces such as DEM files. The contours are created on a separate layer and saved in SDF format so that they can be easily reused in other maps.
The difference in elevation between contour lines is called the contour interval. Usually there are minor contour lines, for example, every ten meters, and major contour lines, perhaps every 100 meters. Small scale maps of regions will have a larger contour interval, and will be less accurate, and large scale maps, suitable for engineering and planning, will have a small contour interval, and be very accurate.
When you choose the contour interval for your map, you will want to generate enough contours to accurately represent the terrain, but not make the contours so dense that they obscure the other features of the map. The illustration below shows a small area of a city with a surface in the background. What would be an appropriate contour interval for this map?
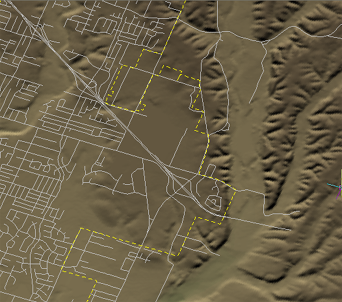
To find out, we can look at the elevation ranges for the surface, which has already been themed by elevation. (For more information, see Change the elevation ranges for a surface.) This tells us that the elevation in the area covered by the map ranges from about 400 meters to just over 700 meters. An interval of one meter would be too dense to be readable, while an interval of 10 meters would be too sparse to be accurate. A little calculation and experimentation shows us that a minor interval of 5 meters and a major interval of 10 meters would give us a good result for this map (see the view on the right in the illustration below).